Understanding the Volume of a Tetrahedron: A Unique Approach
Written on
Chapter 1: Introduction to Tetrahedrons
Calculating the areas of simple 2D shapes like triangles and squares is often a straightforward task. However, determining the volume of 3D shapes, such as tetrahedrons, poses a greater challenge. While we can easily compute the volume of a cube, more complex shapes like icosahedrons require more involved methods.
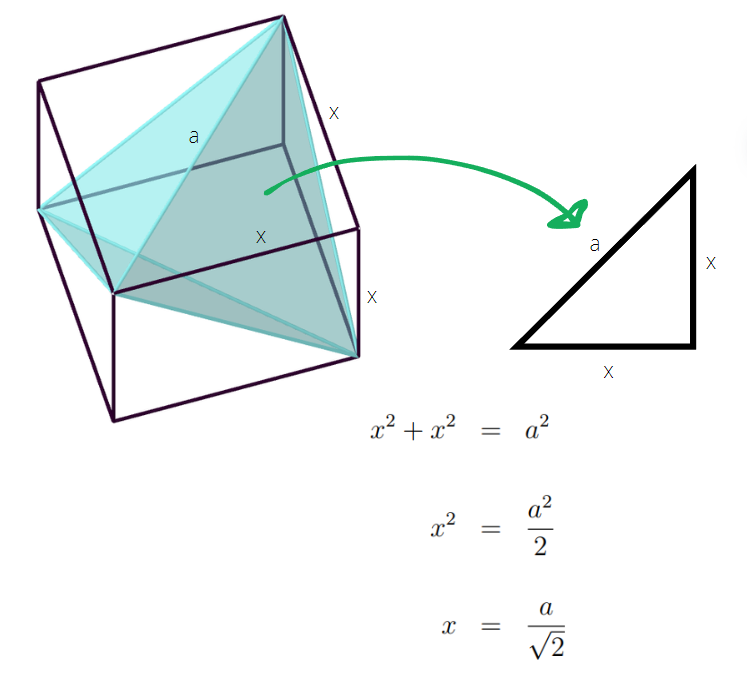
To calculate the volume of a regular tetrahedron — one of the five platonic solids characterized by four triangular faces — we can start by inscribing it within a cube. Let’s assume the tetrahedron has a side length of ( a ).
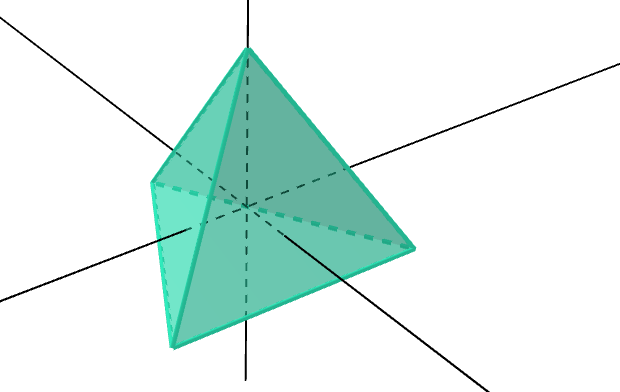
Chapter 2: Geometric Insights
When we inscribe a tetrahedron in a cube, it divides the cube into five distinct regions: the interior of the tetrahedron itself and four pyramids. Below is a visual representation of one such pyramid:
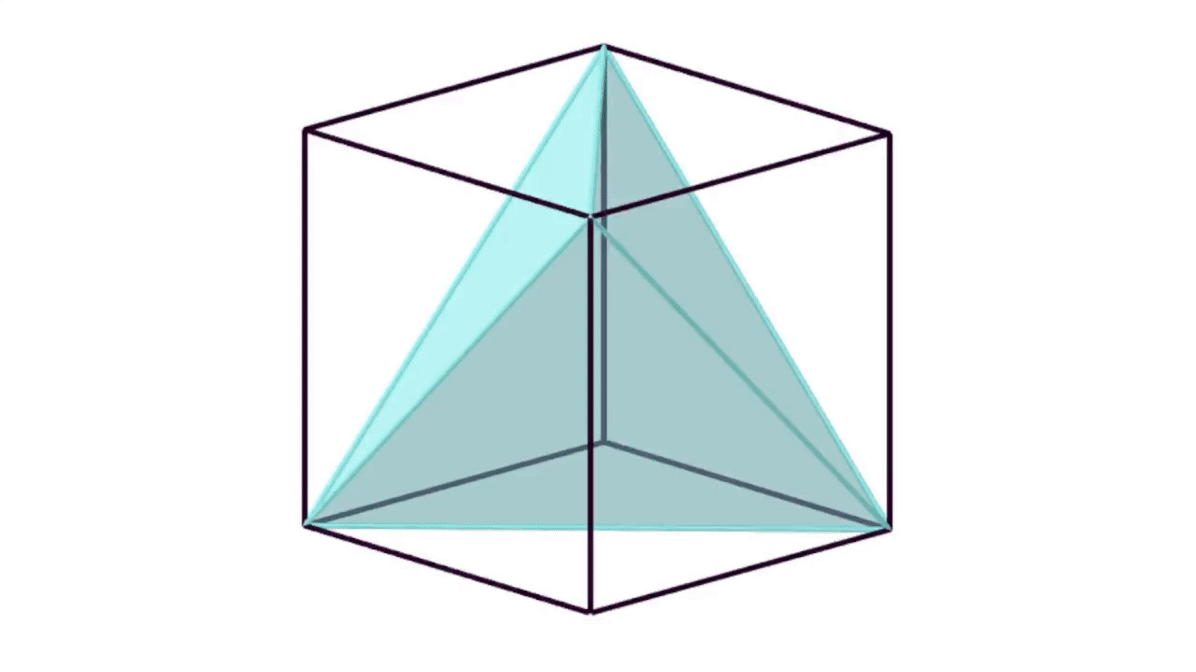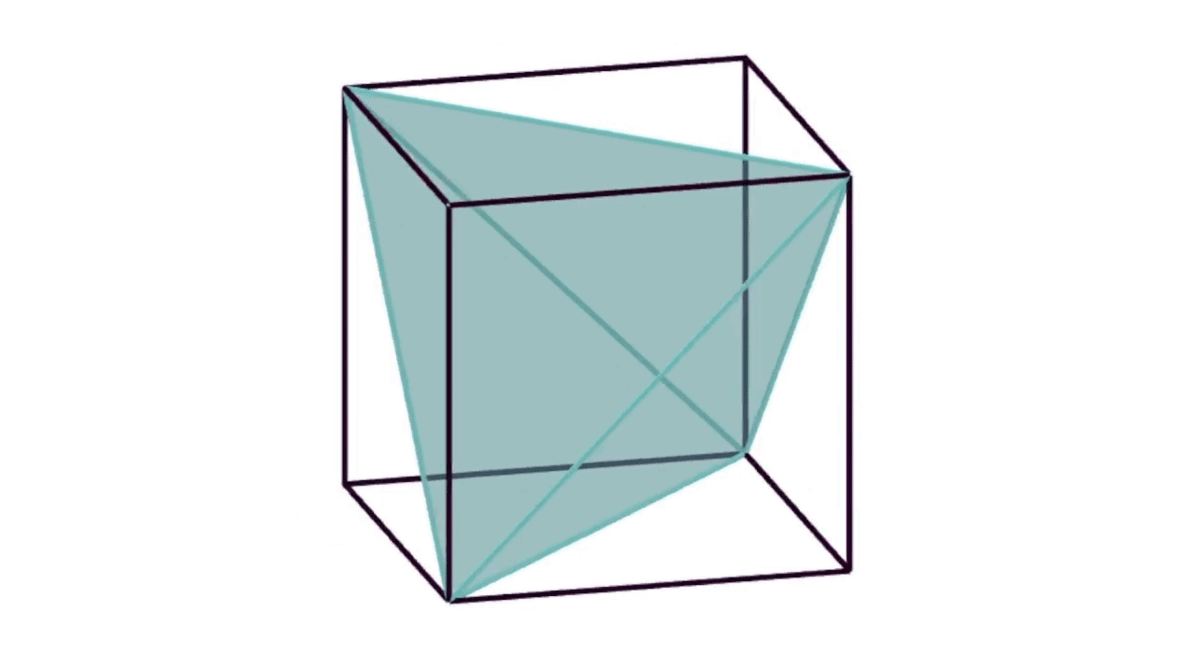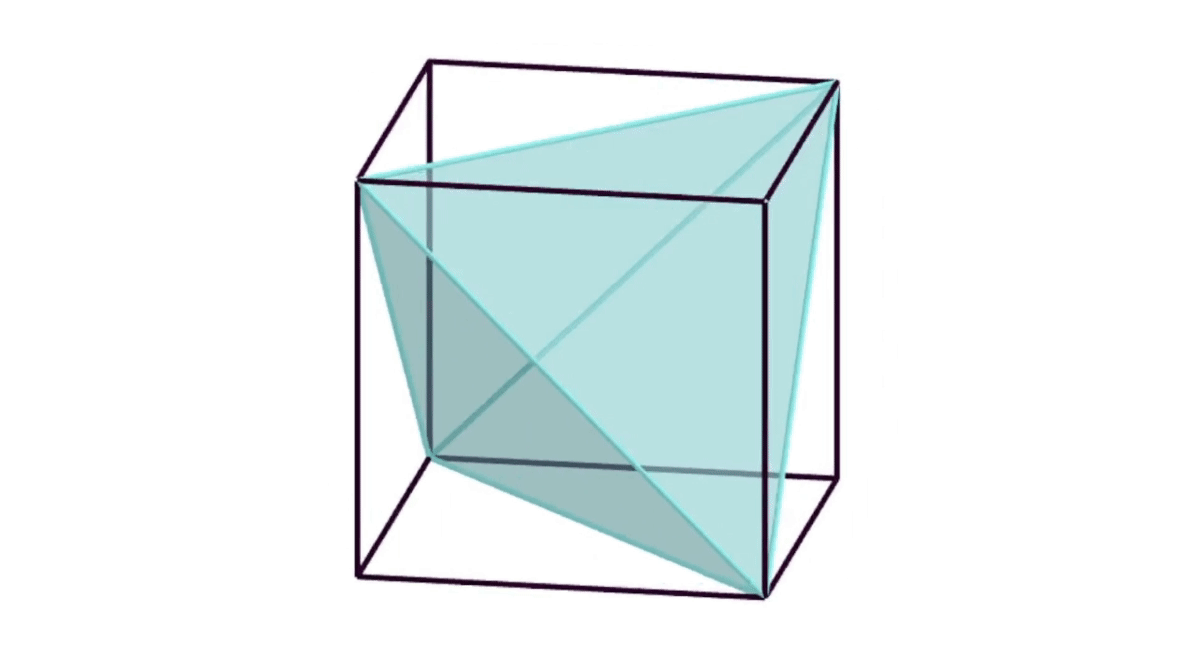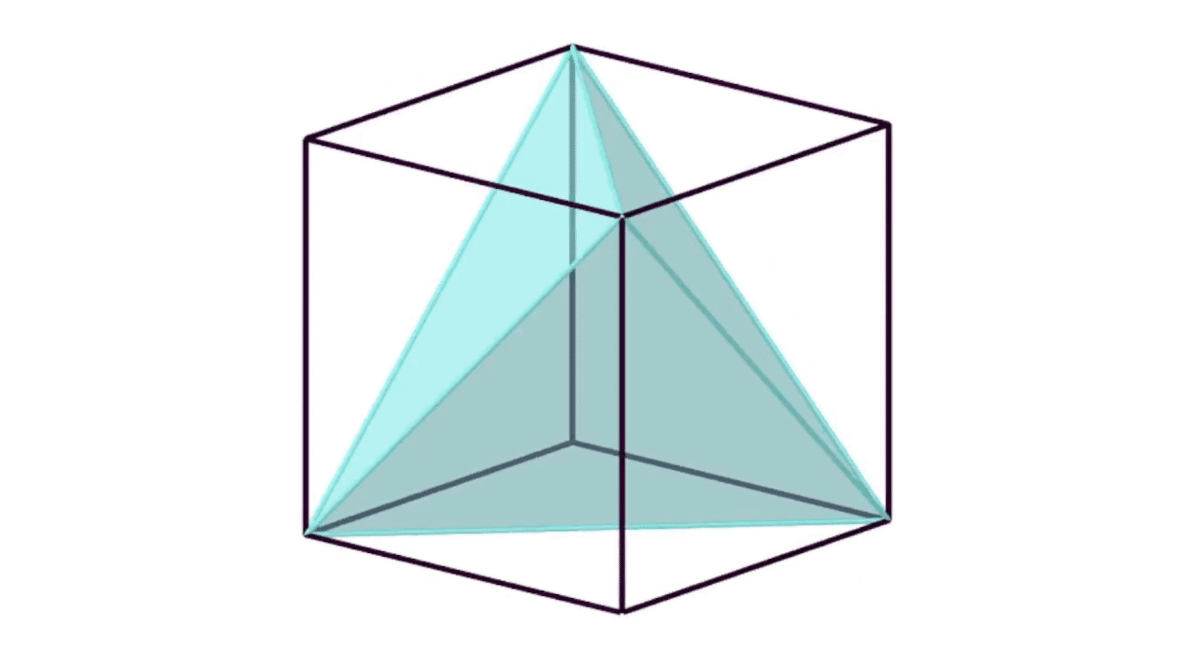
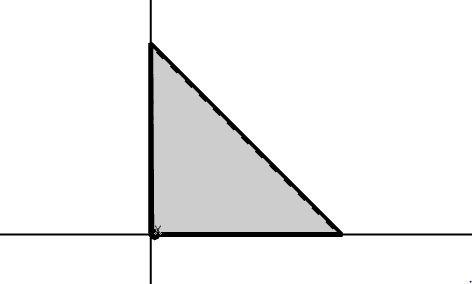
To determine the volume of the tetrahedron, we first need to find the fraction of the cube that one of these pyramids occupies. Let’s consider a cube with a side length of 1. Each side of the pyramid will then also measure 1. Looking at the side profile:

This configuration forms a right triangle. By taking successive cross-sections of this pyramid, we create a series of smaller right triangles:
The side lengths of these triangles decrease from 1 to 0. Conversely, we can think of them as increasing from 0 to 1. If we applied this concept to a 2D triangle, we would observe lines extending from length 0 up to 1.
Imagining these triangles arranged back-to-back leads us to visualize our pyramid. To compute its volume, we must sum the areas of these triangles continuously, which is accomplished through integration.
If we denote the side length of each triangle as ( x ), ranging from 0 to 1, then the area of each triangle can be expressed as ( frac{x^2}{2} ).
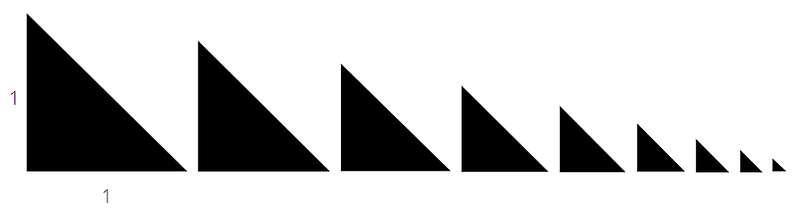
Since the volume of a cube with side length 1 is ( 1^3 = 1 ), it follows that our pyramid occupies ( frac{1}{6} ) of the cube. Remember, a tetrahedron inscribed in a cube occupies the remaining volume after accounting for four pyramids. Thus, it occupies ( 1 - frac{4}{6} = frac{2}{6} = frac{1}{3} ) of the cube.
For a tetrahedron with side length ( a ), we can reassess the tetrahedron-cube configuration to determine the side length of the cube:
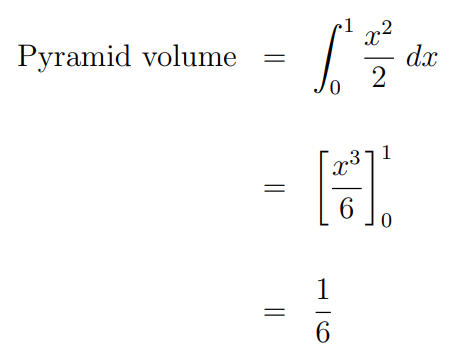
The volume of the cube can be expressed as:
Since the tetrahedron occupies ( frac{1}{3} ) of the cube, we arrive at the formula we sought:
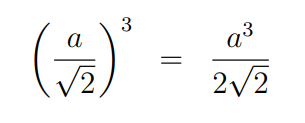
This method, while not the only approach, is one of the most elegant. It beautifully combines geometry, calculus, and Pythagorean principles.
Keep an eye out for future discussions on calculating the volumes of dodecahedrons and icosahedrons!
A complete explanation of the volume of a tetrahedron.
Understanding the height and volume of a regular tetrahedron.